Promiscuous Collisons [_v2]

Morphed Geometry

As I continued experimenting with Grasshopper, I was concentrating on using my existing surfaces from the motion study and applying a geometry to it. The last attempt used a surface that resulted in a fish-scale looking surface, and this time I used a sphere as my original geometry. These choices were more random than studied, yet the output was still intriguing  and allowed me to study how Grasshopper para metrically controls geometry.

definitions for each surface

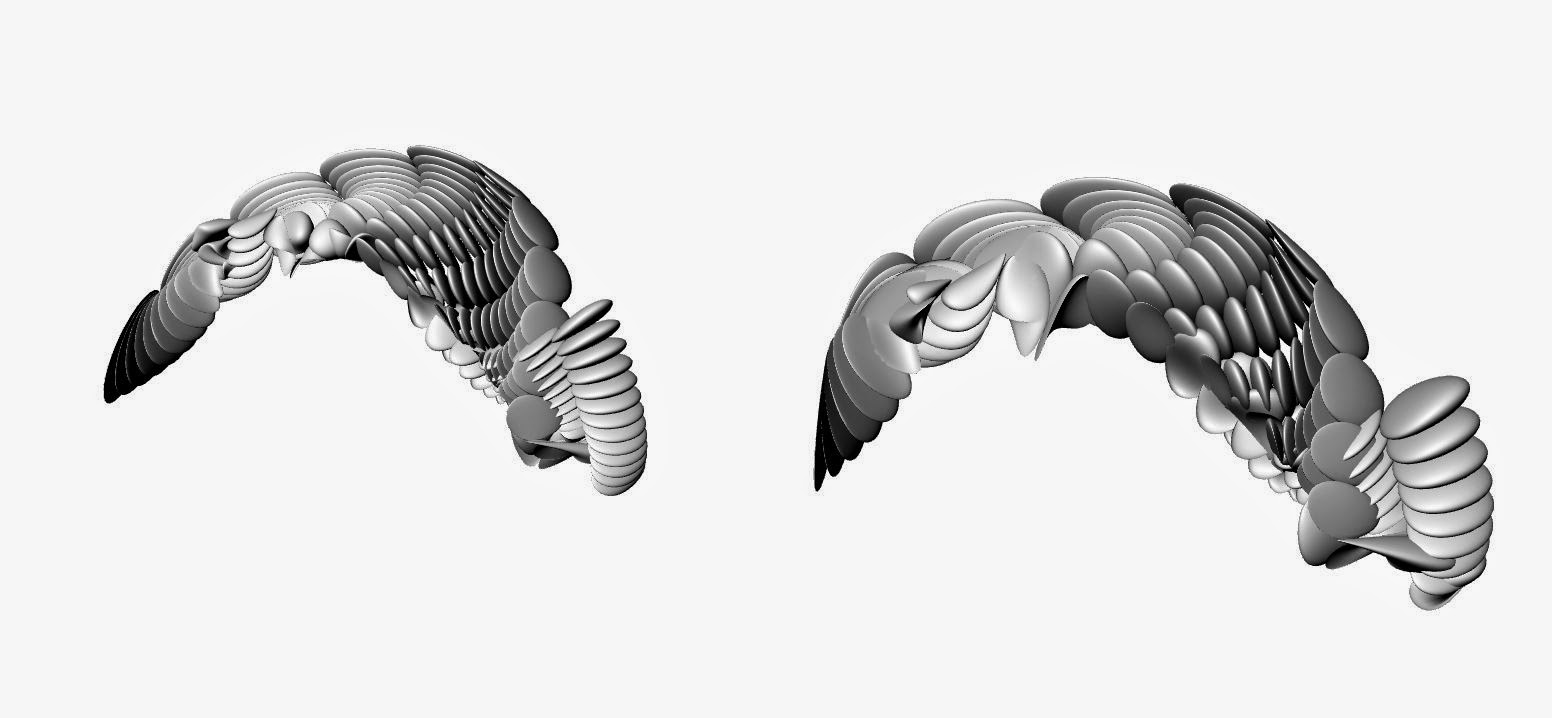
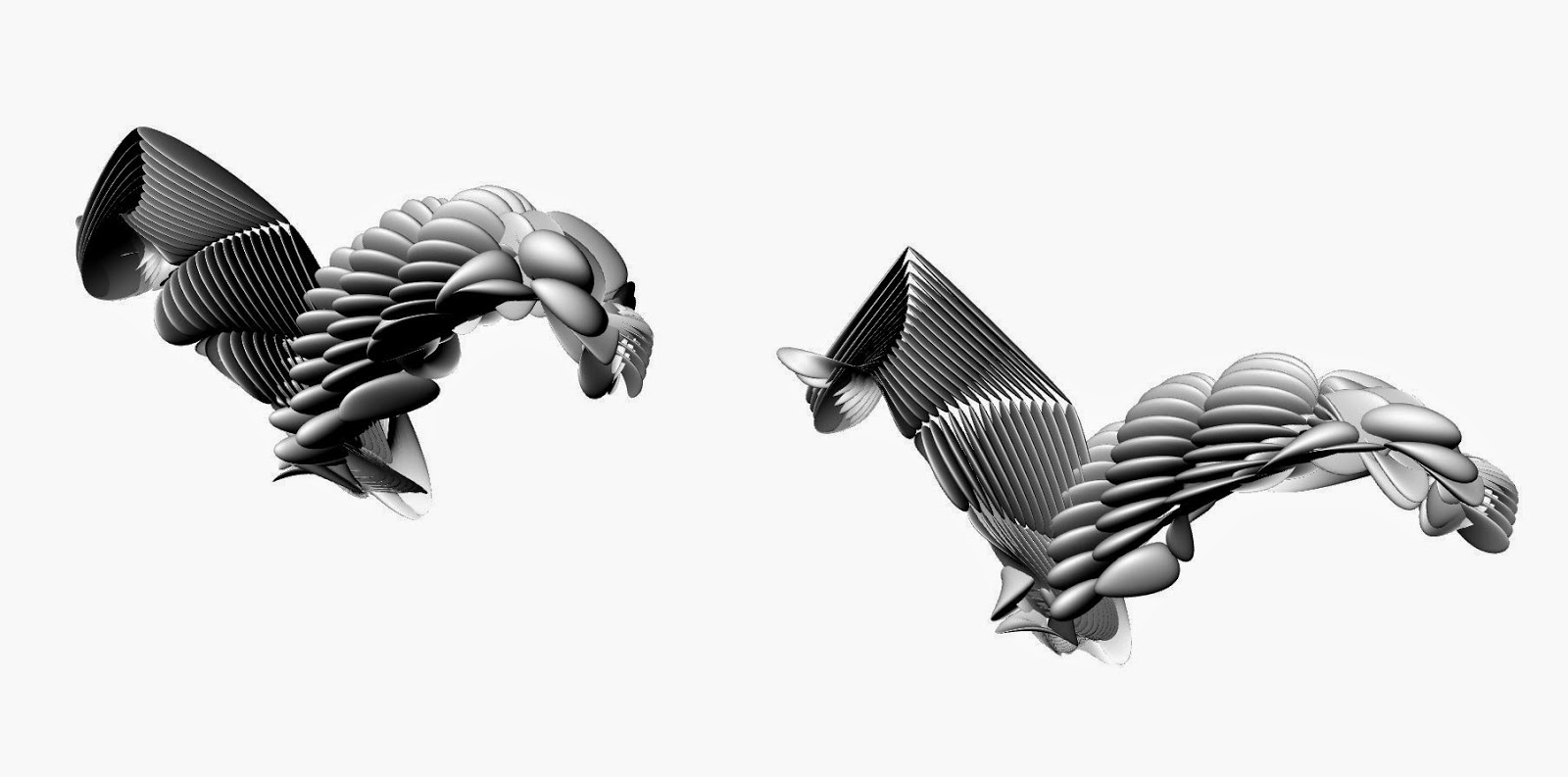
Iterations of the morphed geometry surface

As seen in the image above, I captured moments of adjusting the sliders that controlled the U and V values for the division of the surface.

In these two iterations, the left surface has more divisions than the right. 

In these two iterations, the adjustments I made were of the direction of the geometry, either above the surface or below.

What was interesting was that the way in which I created the definition, the surface was divided in even increments regardless of the geometry of the surface, rather than being based on the geometry of the surface. This resulted in the applied geometry to be morphed unevenly to the surface.  It is almost a battle of geometry. There is: 1) the geometry of the motion surface, which is more complex in some spots than in others, 2) the geometry in how the surface is divided in the U and V direction, and 3) the geometry of the applied object and how it reacts to the divided surface.

Promiscuous Collisions [_v1]

Project 2b: Initial Attempts at Grasshopper

This project is my first foray into the world of Grasshopper. After watching some tutuorials, I tried to attempt to create a definition that subdivides a surface and applies it with a morphed geometry. I have used one of the surfaces of my original motion of a cartwheel as a base for this exploration. 

I created a simple lofted geometry in Rhino that I applied to the surface of motion. Using sliders, I was able to control the size of the applied geometry as well as the amounts of divisions within the surface.

exploration of surface manipulation

series of morphed geometry with original surface

While this exercise helped my familiarity with the tool, I quickly realized while working that this does not relate to the concepts of time in space/ space in time that we are examining.  I realized I needed to re-examine the conceptual agenda of my project to get an idea of what I wanted to accomplish with a parametric tool. This cartoon explores my thoughts of what I would like to examine within the study of the motion of a cartwheel. The motion is about both a combination of limbs moving individually to form an overall holistic movement and the irregularity that exists in what seems like a cyclical motion.

My next attempt with Grasshopper is to parametrically control a surface with data points or curves from the surfaces I created that capture the motion of a cartwheel in space and time.

Project 2a- Animation

Surfaces of Motion

From the contours of motion studied, I generated surfaces of individual limbs, then compiled them to create a three dimensional component to the 2D drawing.

Individual surfaces

perspective view of overlapped surfaces

 

Front view wireframe

Virtual Projection

Re-presenting motion of the human body

motion of study: the cartwheel

composition of frames of video

motion of right arm

motion of left arm

motion of right leg

motion of left leg

Beginning to examine contours of motion without context of human body:

Versioning

response to Versioning: Evolutionary Techniques in Architecture

SHoP

SHoP begins their introduction by providing a definition for versioning as "an operative term to describe recent, significant shift in the way architects and designers are using technology to expand... the potential effects of design on our world." I appreciated their introduction as it cut to the chase and provided a robust overview of this idea of 'versioning'. Before this reading, I was not exposed to this ideology that has formed from current technological advancements, or as SHop put it, "an attitude not ideology." 

The idea of testing and adapting sets of conditions is similar to Angelil's view of education as research oriented-- designed by probing questions and challenging norms. SHoP views this approach as a "process product" where the focus is on the fabrication process rather than a final image, also similar to Angelil's critique of architecture's focus on object. "Here the form, the forces that shape it, and the assemblage of materials in which we execute the ideology are part of the same gesture."

SHoP's Dunescape at MoMA PS1

Office dA

Monica Ponce de Leon and Nader Tehrani

Representing their approach to versioning as a reciprocal and codependent relationship between surface and space allows the exploration of the relationship between geometry and tectonic systems. Their goal is to have the skin and interior space(s) of buildings to read as one, rather than two separate systems. For most of architectural history, these two can be seen as separate systems, as discussed, such as load-bearing Renaissance construction in which the facade is read as an applique pasted onto a heavy building mass. 

"Precision becomes the operative technique for manipulating the membrane that materialises or represents the contents of the spaces of buildings." This idea was illustrated through Kahn's Exeter Library and Eladio Dieste's Church of Christ the Worker. Kahn uses the unit of a brick as patterning, but it becomes a system independent of the structural system, whereas Dieste combines surface and space into a singular entity, yet the geometry of the tectonic units is sacrificed. Geometry and patterning are in opposition in both of these works. Office dA attempts to address these simultaneously, such as the example they give of  their Hookah bar at Mantra. Plywood 'bricks' are employed as the unit of structure. This might simplify the relationship between geometry and tectonics, but it complicates its reading. The beauty I see in Dieste's Church is the advanced geometry used to create a skin simplistic in materiality yet rich in character and experience.

Compositional Surfaces

For 1c, I examined the matrix that I have been working on and was interested in the surface that looked like  frayed ribbon, with curling edges.

I selected the last three surfaces from the series of morphologies of the original object to further study the geometries of surfaces. From these three surfaces, I chose the end segment (in red) to isolate. 

I then isolated the pieces from their parent surfaces, rotating them and seeing how they morphed from one to the next.

When I turned on the control points for the surfaces, I was surprised to find the control points for the entire original surface embedded in the segments. 

Below are the surfaces I am 3D printing. I want to see, physically, how the added complexity in control points affects the surface as it morphs.

Physical manifestation of surfaces